

§U§@≠∂: Extension Field
§W§@≠∂: Ωu© •Nº∆™∫¿≥•Œ
´e§@≠∂: Ωu© •Nº∆∞Ú•ª© ΩË.
ß⁄≠Ã≠∫•˝®”¨ð§@®«®“§l,
•B≠p∫‚®‰ dimension.
∞≤≥] F ¨O§@≠” field, ß⁄≠ö“º F[x] ≥o§@≠” polynomial ring.
´ÐÆe©ˆ¨ð•X®” F[x] ©M F ∫°®¨ Definition 9.3.1 §§ (VS1) ®Ï
(VS4) © ΩË, ¨G™æ F[x] ¨O§@≠” vector space over F. ¶Ð©Û F[x]
∑|§£∑|¨O finite dimensional vector space over F ©O?
Proposition 9.3.5
∞≤≥]
F ¨O§@≠” field, ≠Y±N
F[
x] ¨ð¶®¨O§@≠” vector space over
F,
´h
F[
x] §£¨O finite dimensional vector space over
F.
µ˝ ©˙.
ß⁄≠ÃßQ•Œ§œ√“™k. ∞≤≥]
F[
x] ¨O finite dimensional over
F •B
dim
F(
F[
x]) =
n, ´h¶“º
1,
x,
x2,...,
xn  F
F[
x], ß⁄≠Ã≠n≈Á√“
1,
x,
x2,...,
xn ¨O linearly independent over
F.
≥o¨O¶]¨∞πÔ•Ù∑N§£•˛¨∞ 0 ™∫
c0,
c1,...,
cn ß⁄≠Ùæ
c0 . 1 +
c1 . x +
... +
cn . xn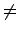
0.
™`∑N
1,
x,
x2,...,
xn ¶@¶≥
n + 1 ≠”§∏Ø¿, ¨GßQ•Œ Lemma
9.3.4 (2) ™æ
n + 1
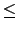
dim
F(
F[
x]) =
n,
¶]¶”±o®Ï•Ÿ¨Þ. ©“•H
F[
x]
§£•iØý¨O finite dimensional over
F.

±µµ€ß⁄≠ö“º•t§@≠” ring. ∞≤≥]
f (x)  F[x] •B
deg(f (x))
F[x] •B
deg(f (x))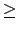 1,
ß⁄≠ö“º
R = F[x]/
1,
ß⁄≠ö“º
R = F[x]/ f (x)
f (x) ≥o§@≠” quotient ring. ¶^≈U§@§U R
§§™∫§∏Ø¿≥£¨O
≥o§@≠” quotient ring. ¶^≈U§@§U R
§§™∫§∏Ø¿≥£¨O
 ™∫ߌ¶°, ®‰§§
g(x)
™∫ߌ¶°, ®‰§§
g(x)  F[x]. πÔ•Ù∑N™∫ c
F[x]. πÔ•Ù∑N™∫ c  F,
F,

 R, ß⁄≠éw∏q
R, ß⁄≠éw∏q
c . 
=
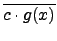
.
≥o≠”πB∫‚¨O well-defined. ¶]¨∞≠Y
 =
= 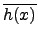 ™Ì•Ð,
g(x) - h(x)
™Ì•Ð,
g(x) - h(x) 
 f (x)
f (x) . §S¶]¨∞
c
. §S¶]¨∞
c  F
F  F[x] •B
F[x] •B
 f (x)
f (x) ¨O F[x] ™∫§@≠” ideal, ß⁄≠Ã∑̵M¶≥
c . (g(x) - h(x))
¨O F[x] ™∫§@≠” ideal, ß⁄≠Ã∑̵M¶≥
c . (g(x) - h(x)) 
 f (x)
f (x) , ¨G™æ
c .
, ¨G™æ
c .  = c .
= c . 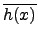 . ßQ•Œ≥o≠” F πÔ R
™∫πB∫‚ß⁄≠ôÐÆe©ˆ≈Á√“ R ¨O§@≠” vector space over F. ®∫ªÚ R
∑|§£∑|¨O finite dimensional vector space over F ©O?
. ßQ•Œ≥o≠” F πÔ R
™∫πB∫‚ß⁄≠ôÐÆe©ˆ≈Á√“ R ¨O§@≠” vector space over F. ®∫ªÚ R
∑|§£∑|¨O finite dimensional vector space over F ©O?
Lemma 9.3.6
∞≤≥]
F ¨O§@≠” field, ≠Y
f (
x)
 F
F[
x] •B
deg(
f (
x))
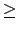
1, ´h
R =
F[
x]/
 f
f (
x)

≥o§@≠” quotient ring ¨O§@≠” finite dimensional
vector space over
F ¶”•B
dim
F(
R) = deg(
f (
x)).
µ˝ ©˙.
∞≤≥]
deg(
f (
x)) =
n, ß⁄≠Ã≠n√“©˙
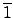
,
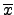
,...,
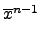
 R
R ¨O
R over
F ™∫§@≤’ basis.
≠∫•˝√“©˙
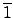 ,
,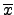 ,...,
,...,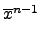 span R over F. •Ù®˙
span R over F. •Ù®˙

 R, ®‰§§
g(x)
R, ®‰§§
g(x)  F[x], ß⁄≠Ã≠n߉®Ï
c0, c1,..., cn - 1
F[x], ß⁄≠Ã≠n߉®Ï
c0, c1,..., cn - 1  F ®œ±o
F ®œ±o

=
c0 . 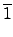
+
c1 . 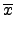
+
... +
cn - 1 . 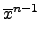
.
•— Theorem
7.2.4, ß⁄≠ÙæπD¶s¶b
h(
x),
r(
x)
 F
F[
x] ∫°®¨
g(
x) =
f (
x)
. h(
x) +
r(
x), ®‰§§
r(
x) = 0 ©Œ
deg(
r(
x)) < deg(
f (
x)). ¶]¨∞
g(
x) -
r(
x) =
f (
x)
. h(
x)

 f
f (
x)

, •— quotient ring ™∫©w∏q™æ

=
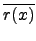
. ≤≠Y
r(
x) = 0, ™æ

=
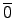
, ¨G®˙
c0 =
c1 =
... =
cn - 1 = 0 Æ…•i±o

=
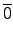
=
c0 . 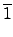
+
c1 . 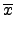
+
... +
cn - 1 . 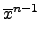
.
•t§@§Ë≠±≠Y
r(
x)

0, ´h•—
deg(
r(
x))
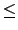 n
n - 1 ™æ¶s¶b
a0,
a1,...,
an - 1  F
F ®œ±o
r(
x) =
a0 +
a1x +
... +
an - 1xn - 1, ¨G•O
c0 =
a0,...,
cn - 1 =
an - 1 Æ…ß⁄≠ö≥

=
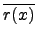
=
c0 . 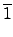
+
c1 . 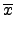
+
... +
cn - 1 . 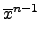
.
©“•H
R
§§™∫§∏Ø¿≥£•i•—
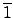
,...,
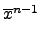
span over
F ±o®Ï.
±µµ€√“©˙
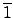 ,
,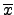 ,...,
,...,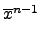 ¨O linearly independent
over F. ß⁄≠ÃßQ•Œ§œ√“™k. ∞≤≥]¶s¶b§£•˛¨∞ 0 ™∫
c0, c1, ... , cn - 1
¨O linearly independent
over F. ß⁄≠ÃßQ•Œ§œ√“™k. ∞≤≥]¶s¶b§£•˛¨∞ 0 ™∫
c0, c1, ... , cn - 1  F ®œ±o
F ®œ±o
c0 . 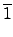
+
c1 . 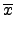
+
... +
cn - 1 . 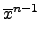
=
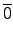
,
™Ì•Ð
g(
x) =
c0 +
... +
cn - 1xn - 1 ≥o≠”´D 0 ™∫¶h∂µ¶°≤≈¶X

=
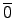
. ¥´•y∏Ъ°
g(
x)

 f
f (
x)

. ¶]
g(
x)

0,
¨G™æ¶s¶b
h(
x)
 F
F[
x] •B
h(
x)

0 ®œ±o
g(
x) =
f (
x)
. h(
x).
∆[πÓ degree ™æ
deg(
g(
x)) = deg(
f (
x)) + deg(
h(
x))
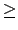
deg(
f (
x)) =
n,
§£πL•—∑Ì™Ï
g(
x) ™∫øÔ®˙, ß⁄≠ÙæπD
deg(
g(
x))
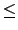 n
n - 1, ¶]¶π±o®Ï•Ÿ¨Þ. ¨G™æ
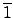
,
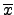
,...,
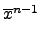
¨O linearly independent over
F.
ß⁄≠çw√“±o
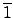 ,
,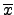 ,...,
,...,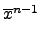
 R ¨O R over F
™∫§@≤’ basis. §S¶]
R ¨O R over F
™∫§@≤’ basis. §S¶]
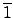 ,
,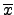 ,...,
,...,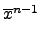 §§¶@¶≥ n
≠”§∏Ø¿, ¨G™æ
dimF(R) = n = deg(f (x)).
§§¶@¶≥ n
≠”§∏Ø¿, ¨G™æ
dimF(R) = n = deg(f (x)). 
∑Ì R ¨O§@≠” integral domain •B F ¨O§@≠”•]ßt©Û R ™∫ field Æ…,
ß⁄≠ç]•i•H±N R ¨ð¶®¨O§@≠” vector space over F. ®∆πͧW•— ring
™∫© ΩË•[§W
F  R, Definition 9.3.1 §§™∫ (VS1), (VS2)
•H§Œ (VS3) ¶€µM≥£≤≈¶X, ß⁄≠Ã∞þ§@≠n¿À¨d™∫¨O (VS4). ∞≤≥] 1F, 1R
§¿ßO¨O F ©M R ≠º™k™∫ identity, ß⁄≠Õu≠n¿ÀπÓ 1F = 1R ßY•i.
≥o¨O¶]¨∞ (VS4) ¨Oª°πÔ•Ù∑N™∫ a
R, Definition 9.3.1 §§™∫ (VS1), (VS2)
•H§Œ (VS3) ¶€µM≥£≤≈¶X, ß⁄≠Ã∞þ§@≠n¿À¨d™∫¨O (VS4). ∞≤≥] 1F, 1R
§¿ßO¨O F ©M R ≠º™k™∫ identity, ß⁄≠Õu≠n¿ÀπÓ 1F = 1R ßY•i.
≥o¨O¶]¨∞ (VS4) ¨Oª°πÔ•Ù∑N™∫ a  R ≠n≤≈¶X
1F . a = a.
¶]¶π≠YØý√“±o 1F = 1R, ®∫ªÚ§W¶°¶€µM¶®•þ. ≠n™`∑Nß⁄≠Ã¥ø∏g¨ðπL®“§l§@≠”
subring ™∫ identity §£§@©w∑|¨O≠Ï®”™∫ ring ™∫ identity. §£πL•—©Û≤¶b
R ¨O integral domain, ®∆±°¥N®S¶≥®∫ªÚΩ∆¬¯§F. ß⁄≠Õu≠n•Ù®˙ F
§§™∫§@≠”´D 0 §∏Ø¿ c, ±N•¶¶“º¶®¨O F ™∫§∏Ø¿, ß⁄≠ö≥
1F . c = c; •t§@§Ë≠±±N•¶¨ð¶®¨O R ™∫§∏Ø¿, ß⁄≠ö≥
1R . c = c.
µ≤¶X§W≠±®‚≠”µ•¶°±o:
(1F - 1R) . c = 0. •—©Û R ¨O integral
domain •B c
R ≠n≤≈¶X
1F . a = a.
¶]¶π≠YØý√“±o 1F = 1R, ®∫ªÚ§W¶°¶€µM¶®•þ. ≠n™`∑Nß⁄≠Ã¥ø∏g¨ðπL®“§l§@≠”
subring ™∫ identity §£§@©w∑|¨O≠Ï®”™∫ ring ™∫ identity. §£πL•—©Û≤¶b
R ¨O integral domain, ®∆±°¥N®S¶≥®∫ªÚΩ∆¬¯§F. ß⁄≠Õu≠n•Ù®˙ F
§§™∫§@≠”´D 0 §∏Ø¿ c, ±N•¶¶“º¶®¨O F ™∫§∏Ø¿, ß⁄≠ö≥
1F . c = c; •t§@§Ë≠±±N•¶¨ð¶®¨O R ™∫§∏Ø¿, ß⁄≠ö≥
1R . c = c.
µ≤¶X§W≠±®‚≠”µ•¶°±o:
(1F - 1R) . c = 0. •—©Û R ¨O integral
domain •B c 0, ©“•Hß⁄≠ö≥ 1F = 1R.
0, ©“•Hß⁄≠ö≥ 1F = 1R.
¨JµM R ¨O§@≠” over F ™∫ vector space, ß⁄≠ð∑Ì R ¨O finite
dimensional over F Æ…•¶¶≥§∞ªÚ≠´≠nØS© .
Theorem 9.3.7
∞≤≥]
R ¨O§@≠” integral domain,
F ¨O§@≠” field •B
F  R
R.
§S∞≤≥]
R ¨ð¶®¨O§@≠” vector space over
F Æ…¨O finite dimensional
over
F, ´h
- πÔ•Ù∑N a
 R, ¨“¶s¶b§@≠”´D 0 ™∫
f (x)
R, ¨“¶s¶b§@≠”´D 0 ™∫
f (x)  F[x] ®œ±o f (a) = 0.
F[x] ®œ±o f (a) = 0.
- R ¨O§@≠” field.
µ˝ ©˙.
ß⁄≠Ã∞≤≥]
dim
F(
R) =
n.
(1) ¶“º
1, a, a2,..., an ≥o n + 1 ≠” R §§™∫§∏Ø¿. ¶p™G•¶≠èO
linearly independent over F, ´h•— Lemma 9.3.4 (2) ±o
n = dim
F(
R)
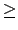 n
n + 1,
≥y¶®•Ÿ¨Þ, ¨G™æ
1,
a,
a2,...,
an §£¨O linearly independent over
F.
¥´•y∏Ъ°¶s¶b§£•˛¨∞ 0 ™∫
c0,
c1,...,
cn  F
F, ∫°®¨
c0 . 1 + c1 . a + ... + cn . an = 0.
¨G•O
f (
x) =
c0 +
c1x +
... +
cnxn, ß⁄≠ño
f (
x)

0 •B
f (
a) = 0.
(2) ¶] R §w™æ¨O integral domain, ≠n√“©˙ R ¨O§@≠” field,
ß⁄≠Õu≠n√“©˙ R §§§£¨∞ 0 ™∫§∏Ø¿≥£¨O unit. ¥´•y∏Ъ°≠n√“©˙πÔ•Ù∑N
a  R •B a
R •B a 0, ¨“¶s¶b b
0, ¨“¶s¶b b  R ∫°®¨
a . b = 1. •— (1)
™æ¶s¶b´D 0 ™∫¶h∂µ¶° f (x) ∫°®¨ f (a) = 0. ß⁄≠Ã∞≤≥]
R ∫°®¨
a . b = 1. •— (1)
™æ¶s¶b´D 0 ™∫¶h∂µ¶° f (x) ∫°®¨ f (a) = 0. ß⁄≠Ã∞≤≥]
f (
x) =
c0 +
c1x +
... +
cmxm 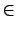 F
F[
x]
¨O
F[
x] §§´D 0 •B∫°®¨
f (
a) = 0 ™∫ degree ≥çp™∫ polynomial. •— degree ≥çp™∫∞≤≥], ß⁄≠Õi±o
c0
0. ≥o¨O¶]¨∞≠Y
c0 = 0, ´h•—
f (a) = c1 . a + ... + cm . am = (c1 + c2 . a + ... + cm . am - 1) . a = 0
•H§Œ
R ¨O integral domain ±o
g(
a) = 0, ®‰§§
g(
x) =
c1 +
c2x +
... +
cmxm - 1  F
F[
x] §£¨∞ 0 •B
deg(
g(
x)) < deg(
f (
x)). ¶π©M
f (
x) ¨O degree ≥çp™∫߉™k¨€•Ÿ¨Þ,
¨G±o
c0
0. ≤±N
f (
a) = 0 ™∫
c0 ≤æ¶Ðµ•¶°™∫•t§@√‰, ß⁄≠ño
(c1 + c2 . a + ... + cm . am - 1) . a = - c0.
¶]¶π≠Y•O
b = (- c0)-1 . (c1 + c2 . a + ... + cm . am - 1),
´hß⁄≠ö≥
a . b = 1. ™`∑N•—©Û
-
c0  F
F •B -
c0
0 •H§Œ
F ¨O§@≠” field, ß⁄≠ö≥
(-
c0)
-1  F
F  R
R, ¶A•[§W
c1 +
c2 . a +
... +
cm . am - 1  R
R ß⁄≠ño
b  R
R, ¨G™æ
a ¨O
R
™∫§@≠” unit.

ßQ•Œ Theorem 9.3.7 ß⁄≠Õi•H´Ðß÷™∫µπ Proposition 9.3.5
•t§@≠”√“©˙: ∞≤¶p F[x] ¨O finite dimensional over F, •—©Û F[x]
¨O integral domain ßQ•Œ Theorem 9.3.7 ß⁄≠ño F[x] ∑|¨O§@≠”
field. ¶˝≥o¨O§£•iØý™∫, ¶]¨∞ F[x] §§•u¶≥ degree ¨∞ 0 ™∫§∏Ø¿§ ¨O
unit.


§U§@≠∂: Extension Field
§W§@≠∂: Ωu© •Nº∆™∫¿≥•Œ
´e§@≠∂: Ωu© •Nº∆∞Ú•ª© ΩË.
Administrator
2005-06-18
![]() F[x] •B
deg(f (x))
F[x] •B
deg(f (x))![]() 1,
ß⁄≠ö“º
R = F[x]/
1,
ß⁄≠ö“º
R = F[x]/![]() f (x)
f (x)![]() ≥o§@≠” quotient ring. ¶^≈U§@§U R
§§™∫§∏Ø¿≥£¨O
≥o§@≠” quotient ring. ¶^≈U§@§U R
§§™∫§∏Ø¿≥£¨O
![]() ™∫ߌ¶°, ®‰§§
g(x)
™∫ߌ¶°, ®‰§§
g(x) ![]() F[x]. πÔ•Ù∑N™∫ c
F[x]. πÔ•Ù∑N™∫ c ![]() F,
F,
![]()
![]() R, ß⁄≠éw∏q
R, ß⁄≠éw∏q
![]() ,
,![]() ,...,
,...,![]() span R over F. •Ù®˙
span R over F. •Ù®˙
![]()
![]() R, ®‰§§
g(x)
R, ®‰§§
g(x) ![]() F[x], ß⁄≠Ã≠n߉®Ï
c0, c1,..., cn - 1
F[x], ß⁄≠Ã≠n߉®Ï
c0, c1,..., cn - 1 ![]() F ®œ±o
F ®œ±o
![]() ,
,![]() ,...,
,...,![]() ¨O linearly independent
over F. ß⁄≠ÃßQ•Œ§œ√“™k. ∞≤≥]¶s¶b§£•˛¨∞ 0 ™∫
c0, c1, ... , cn - 1
¨O linearly independent
over F. ß⁄≠ÃßQ•Œ§œ√“™k. ∞≤≥]¶s¶b§£•˛¨∞ 0 ™∫
c0, c1, ... , cn - 1 ![]() F ®œ±o
F ®œ±o
![]() ,
,![]() ,...,
,...,![]()
![]() R ¨O R over F
™∫§@≤’ basis. §S¶]
R ¨O R over F
™∫§@≤’ basis. §S¶]
![]() ,
,![]() ,...,
,...,![]() §§¶@¶≥ n
≠”§∏Ø¿, ¨G™æ
dimF(R) = n = deg(f (x)).
§§¶@¶≥ n
≠”§∏Ø¿, ¨G™æ
dimF(R) = n = deg(f (x)). ![]()
![]() R, Definition 9.3.1 §§™∫ (VS1), (VS2)
•H§Œ (VS3) ¶€µM≥£≤≈¶X, ß⁄≠Ã∞þ§@≠n¿À¨d™∫¨O (VS4). ∞≤≥] 1F, 1R
§¿ßO¨O F ©M R ≠º™k™∫ identity, ß⁄≠Õu≠n¿ÀπÓ 1F = 1R ßY•i.
≥o¨O¶]¨∞ (VS4) ¨Oª°πÔ•Ù∑N™∫ a
R, Definition 9.3.1 §§™∫ (VS1), (VS2)
•H§Œ (VS3) ¶€µM≥£≤≈¶X, ß⁄≠Ã∞þ§@≠n¿À¨d™∫¨O (VS4). ∞≤≥] 1F, 1R
§¿ßO¨O F ©M R ≠º™k™∫ identity, ß⁄≠Õu≠n¿ÀπÓ 1F = 1R ßY•i.
≥o¨O¶]¨∞ (VS4) ¨Oª°πÔ•Ù∑N™∫ a ![]() R ≠n≤≈¶X
1F . a = a.
¶]¶π≠YØý√“±o 1F = 1R, ®∫ªÚ§W¶°¶€µM¶®•þ. ≠n™`∑Nß⁄≠Ã¥ø∏g¨ðπL®“§l§@≠”
subring ™∫ identity §£§@©w∑|¨O≠Ï®”™∫ ring ™∫ identity. §£πL•—©Û≤¶b
R ¨O integral domain, ®∆±°¥N®S¶≥®∫ªÚΩ∆¬¯§F. ß⁄≠Õu≠n•Ù®˙ F
§§™∫§@≠”´D 0 §∏Ø¿ c, ±N•¶¶“º¶®¨O F ™∫§∏Ø¿, ß⁄≠ö≥
1F . c = c; •t§@§Ë≠±±N•¶¨ð¶®¨O R ™∫§∏Ø¿, ß⁄≠ö≥
1R . c = c.
µ≤¶X§W≠±®‚≠”µ•¶°±o:
(1F - 1R) . c = 0. •—©Û R ¨O integral
domain •B c
R ≠n≤≈¶X
1F . a = a.
¶]¶π≠YØý√“±o 1F = 1R, ®∫ªÚ§W¶°¶€µM¶®•þ. ≠n™`∑Nß⁄≠Ã¥ø∏g¨ðπL®“§l§@≠”
subring ™∫ identity §£§@©w∑|¨O≠Ï®”™∫ ring ™∫ identity. §£πL•—©Û≤¶b
R ¨O integral domain, ®∆±°¥N®S¶≥®∫ªÚΩ∆¬¯§F. ß⁄≠Õu≠n•Ù®˙ F
§§™∫§@≠”´D 0 §∏Ø¿ c, ±N•¶¶“º¶®¨O F ™∫§∏Ø¿, ß⁄≠ö≥
1F . c = c; •t§@§Ë≠±±N•¶¨ð¶®¨O R ™∫§∏Ø¿, ß⁄≠ö≥
1R . c = c.
µ≤¶X§W≠±®‚≠”µ•¶°±o:
(1F - 1R) . c = 0. •—©Û R ¨O integral
domain •B c![]() 0, ©“•Hß⁄≠ö≥ 1F = 1R.
0, ©“•Hß⁄≠ö≥ 1F = 1R.
![]() R •B a
R •B a![]() 0, ¨“¶s¶b b
0, ¨“¶s¶b b ![]() R ∫°®¨
a . b = 1. •— (1)
™æ¶s¶b´D 0 ™∫¶h∂µ¶° f (x) ∫°®¨ f (a) = 0. ß⁄≠Ã∞≤≥]
R ∫°®¨
a . b = 1. •— (1)
™æ¶s¶b´D 0 ™∫¶h∂µ¶° f (x) ∫°®¨ f (a) = 0. ß⁄≠Ã∞≤≥]