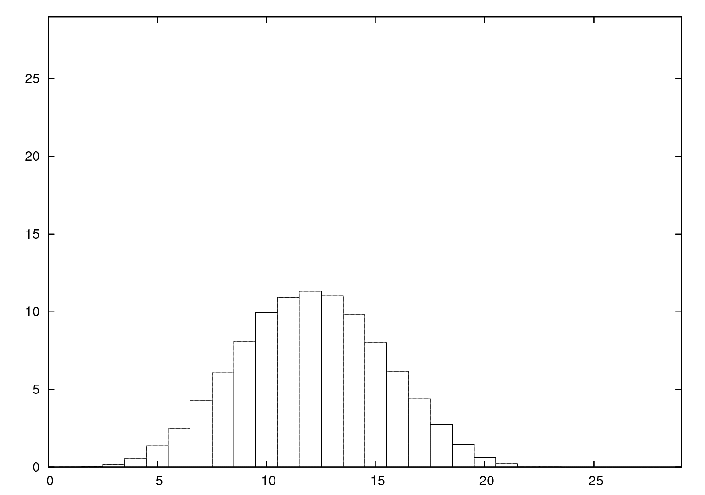
Example for designs in 18 runs
with 6
three-level factors
N=6;
M=18;
Input your prior knowledge on
π1 : the
experimenter's prior belief that the linear term xi
is in the true model
(p1).
π2: the
prior
probability that a quadratic effect is in the true model given that
the
linear effect of the same factor is in the model (p2).
π3:
the
prior probability that an interaction is in the true model given
that the linear
effects of both the factors involved are in the model (p3).
Probabilities for models with
different numbers of parameters being the true
model for a three-level design for six factors (Figure 1 in Tsai et al, 2007 JSPI)
(a) π1=1, π2=1; and π3=0.5
function mod_out.m (called modnom.m,
frac.m)
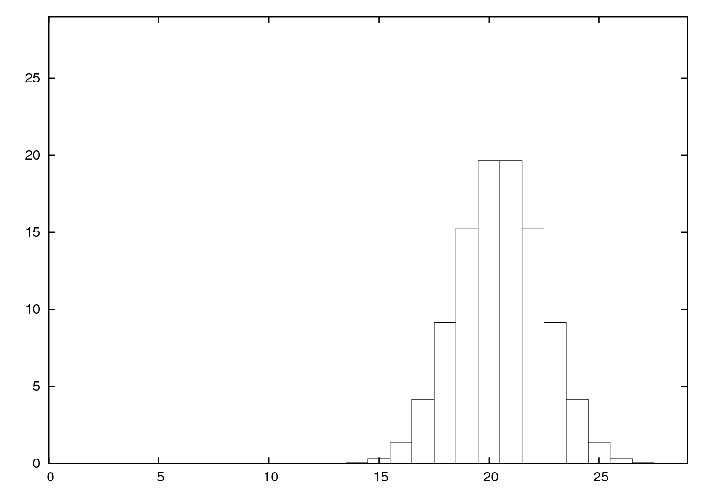
(b)
π1=0.8, π2=0.7 and π3=0.3