

下一頁: The Symmetric Group
上一頁: Finite Abelian Groups
前一頁: Abelian p-groups
既然每一個 finite abelian group 都可寫成一些 abelian p-groups 的
direct product, 而每一個 abelian p-group 也都可寫成一些 cyclic
groups 的 direct product, 因此由這兩個結果我們可以說完整的掌握了
finite abelian groups.
Theorem 3.3.11 (Fundamental Theorem on Finite Abelian Groups)
若
G 是一個 finite abelian group, 則
G 可以寫成一些 cyclic
groups 的 direct product.
証 明.
由 Proposition
3.3.7 知
G 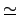 P1
P1×
... ×
Pr,
其中
Pi 都是某個質數
pi 的 abelian
pi-group. 再由
3.3.10 知對所有的
Pi, 都可找到 cyclic groups
Ci1,...
Cini 使得
Pi 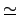 Ci1
Ci1×
... ×
Cini.
因此得證本定理.

這裡很有趣的是我們都知道所有的 cyclic groups 長什麼樣子, 既然 finite
abelian groups 都是 cyclic groups 的 direct product,
我們當然就知道所有的 finite abelian groups 長什麼樣子了. 比方說若
G 是一個 abelian group 且 | G| = 6, 那麼 G 有可能長什麼樣子呢? 由
Proposition 3.3.7 知
G 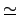 P1×P2 其中 P1 的
order 是 2, P2 的 order 是 3. 而 order 是 2 的 group 一定是
cyclic (Corollary 2.2.3) 故
P1
P1×P2 其中 P1 的
order 是 2, P2 的 order 是 3. 而 order 是 2 的 group 一定是
cyclic (Corollary 2.2.3) 故
P1 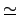
 /2
/2 . 同理
P2
. 同理
P2 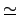
 /3
/3 . 故
G
. 故
G 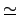
 /2
/2 ×
× /3
/3 . 不過由
3.2.2 知
. 不過由
3.2.2 知
 /2
/2 ×
× /3
/3
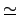
 /6
/6 , 所以
G
, 所以
G 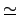
 /6
/6 . 也就是所有的元素個數為 6 的 abelian group 都是
cyclic group. 相信大家不難利用這個例子推廣到以下的 Corollary:
. 也就是所有的元素個數為 6 的 abelian group 都是
cyclic group. 相信大家不難利用這個例子推廣到以下的 Corollary:
Corollary 3.3.12
若
G 是一個 abelian group 且
|
G| =
p1 ... pr 其中
p1,...
pr 是相異的質數, 則
G 是一個 cyclic group.
至於若 G 的 order 的質因數分解中存在高次方的話,
那麼問題就複雜一點了. 例如考慮 order 為 144 的 abelian group G.
因
144 = 24 . 32, 由 Proposition 3.3.7 知
G 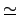 P1×P2 其中 P1 的 order 是 24, P2 的 order 是
32. 再由 Proposition 3.3.10 計算
P1×P2 其中 P1 的 order 是 24, P2 的 order 是
32. 再由 Proposition 3.3.10 計算
4 = 3 + 1 = 2 + 2 = 2 + 1 + 1 = 1 + 1 + 1 + 1
知 P1 有可能是 isomorphic to
(1)

/16

or (2)

/8

×

/2

or (3)

/4

×

/4

,
(4)

/4

×

/2

×

/2

or (5)

/2

×

/2

×

/2

×

/2

,


下一頁: The Symmetric Group
上一頁: Finite Abelian Groups
前一頁: Abelian p-groups
Administrator
2005-06-18
![]() P1×P2 其中 P1 的
order 是 2, P2 的 order 是 3. 而 order 是 2 的 group 一定是
cyclic (Corollary 2.2.3) 故
P1
P1×P2 其中 P1 的
order 是 2, P2 的 order 是 3. 而 order 是 2 的 group 一定是
cyclic (Corollary 2.2.3) 故
P1 ![]()
![]() /2
/2![]() . 同理
P2
. 同理
P2 ![]()
![]() /3
/3![]() . 故
G
. 故
G ![]()
![]() /2
/2![]() ×
×![]() /3
/3![]() . 不過由
3.2.2 知
. 不過由
3.2.2 知
![]() /2
/2![]() ×
×![]() /3
/3![]()
![]()
![]() /6
/6![]() , 所以
G
, 所以
G ![]()
![]() /6
/6![]() . 也就是所有的元素個數為 6 的 abelian group 都是
cyclic group. 相信大家不難利用這個例子推廣到以下的 Corollary:
. 也就是所有的元素個數為 6 的 abelian group 都是
cyclic group. 相信大家不難利用這個例子推廣到以下的 Corollary:
![]() P1×P2 其中 P1 的 order 是 24, P2 的 order 是
32. 再由 Proposition 3.3.10 計算
P1×P2 其中 P1 的 order 是 24, P2 的 order 是
32. 再由 Proposition 3.3.10 計算