在 Gauss's Lemma 中我們需要算出
{a, 2a,...,![]() a}
中有多少元素其除以 p 的餘數大於 (p - 1)/2. 若其個數為 n, 則
a}
中有多少元素其除以 p 的餘數大於 (p - 1)/2. 若其個數為 n, 則
![]()
![]()
![]() = (- 1)n. 由於 (- 1)n 的取值完全取決於 n
是奇數或偶數, 所以我們並不需精確地算出 n 為多少,
只需確認其為奇數或偶數即可. 以下我們將介紹一個判別 n
為奇或偶的方法, 不過由於我們要考慮的
= (- 1)n. 由於 (- 1)n 的取值完全取決於 n
是奇數或偶數, 所以我們並不需精確地算出 n 為多少,
只需確認其為奇數或偶數即可. 以下我們將介紹一個判別 n
為奇或偶的方法, 不過由於我們要考慮的
![]()
![]()
![]() 其中 q 為奇質數,
所以底下的方法中我們僅考慮 a 為奇數的情況.
其中 q 為奇質數,
所以底下的方法中我們僅考慮 a 為奇數的情況.
為了方便我們先介紹一個符號. 給定一實數 r, 我們令 [r]
表示小於等於 r 的整數中最大的整數. 例如若 ![]() 表圓周率, 則
[
表圓周率, 則
[![]() ] = 3. 又例如 [- 5.2] = - 6. 要注意當 m, n 是正整數時 [m/n]
即為 m 除以 n 的商.
] = 3. 又例如 [- 5.2] = - 6. 要注意當 m, n 是正整數時 [m/n]
即為 m 除以 n 的商.
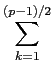 ka =
ka = 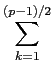 p
p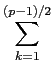 k =
k = 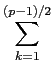
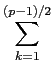
利用 Corollary 5.3.4 以及 Lemma 5.4.2, Lemma
5.4.4, 我們知給定一奇質數 p, 要計算一個奇數 a 其
![]()
![]()
![]() 之值, 我們只要計算
之值, 我們只要計算
![]() [ka/p]
之值即可. 若其值為 N, 則得
[ka/p]
之值即可. 若其值為 N, 則得
![]()
![]()
![]() = (- 1)N. 例如要求
= (- 1)N. 例如要求
![]()
![]()
![]() , 我們只要計算
[5/11] + [10/11] + [15/11] + [20/11] + [25/11]. 算出其值為 4, 故知
, 我們只要計算
[5/11] + [10/11] + [15/11] + [20/11] + [25/11]. 算出其值為 4, 故知
![]()
![]()
![]() = (- 1)4 = 1.
= (- 1)4 = 1.
接著我們要利用 Lemma 5.4.4 來計算
![]()
![]()
![]() . 很容易理解算
. 很容易理解算
![]()
![]()
![]() 不止和 p 有關也和 q 有關, 所以我們要探討
不止和 p 有關也和 q 有關, 所以我們要探討
![]()
![]()
![]() 和
和
![]()
![]()
![]() 的關係. 由於 p, q 皆為奇質數,
我們都可以利用 Lemma 5.4.4 來計算
的關係. 由於 p, q 皆為奇質數,
我們都可以利用 Lemma 5.4.4 來計算
![]()
![]()
![]() 和
和
![]()
![]()
![]() . 因此我們要探討
. 因此我們要探討
![]() [kq/p] 和
[kq/p] 和
![]() [lp/q] 之間的關係.
[lp/q] 之間的關係.
在探討此問題前, 我們再從另一個角度來看 [r] 這個整數. 當 r
是正的實數時, [r] 之值就是所有滿足
0![]() n
n![]() r 的正整數 n
的個數. 在座標 xy-平面上, 我們稱 x-軸及
y-軸座標皆為正整數的點為``正格子點''. 依此看法, 當 k 是正整數時,
[kq/p] 之值就是直線 x = k 在
0
r 的正整數 n
的個數. 在座標 xy-平面上, 我們稱 x-軸及
y-軸座標皆為正整數的點為``正格子點''. 依此看法, 當 k 是正整數時,
[kq/p] 之值就是直線 x = k 在
0![]() y
y![]() kq/p 之間的正格子點個數.
而當 l 是正整數時, [lp/q] 之值就是直線 y = l 在
0
kq/p 之間的正格子點個數.
而當 l 是正整數時, [lp/q] 之值就是直線 y = l 在
0![]() x
x![]() lp/q
之間的正格子點個數. 利用這種觀點, 我們有以下之結果.
lp/q
之間的正格子點個數. 利用這種觀點, 我們有以下之結果.
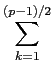
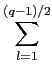

在 T 中的任意正格子點 (m, n), 依定義需滿足
m, n ![]()
![]() 且
0
且
0![]() m
m![]() p/2 及
0
p/2 及
0![]() n
n![]() q/2. 因此由 p, q 為奇數知在 T
中的正格子點個數為
q/2. 因此由 p, q 為奇數知在 T
中的正格子點個數為
![]()
![]() .
.
另一方面在 T1 中的正格子點 (k, s), 依定義需滿足
k, s ![]()
![]() 且
0
且
0![]() k
k![]() p/2 及
0
p/2 及
0![]() s
s![]() kq/p. 也就是說
k
kq/p. 也就是說
k ![]()
![]() 需滿足
1
需滿足
1![]() k
k![]() (p - 1)/2, 且給定 k, 則
0
(p - 1)/2, 且給定 k, 則
0![]() s
s![]() kq/p. 換句話說要計算在
T1 中的格子點, 等於在計算給定
k
kq/p. 換句話說要計算在
T1 中的格子點, 等於在計算給定
k ![]()
![]() 且
1
且
1![]() k
k![]() (p - 1)/2 時
會有多少
s
(p - 1)/2 時
會有多少
s ![]()
![]() 滿足
0
滿足
0![]() s
s![]() kq/p, 再將所有 k
所算得之結果加起來. 然而對任意的正整數 k 符合
0
kq/p, 再將所有 k
所算得之結果加起來. 然而對任意的正整數 k 符合
0![]() s
s![]() kq/p
的正整數 s 的個數為 [kq/p]. 所以在 T1 中的正格子點數為
kq/p
的正整數 s 的個數為 [kq/p]. 所以在 T1 中的正格子點數為
![]() [kq/p]. 同理在 T2 中的正格子點數為
[kq/p]. 同理在 T2 中的正格子點數為
![]() [lp/q].
[lp/q].
在 T1 和 T2 的交界, 即滿足 y = (q/p)x 且
0![]() x
x![]() p/2
的線段上會不會有正格子點呢? 若 (m, n) 為其上之ㄧ正格子點, 則我們有
pn = qm 且
1
p/2
的線段上會不會有正格子點呢? 若 (m, n) 為其上之ㄧ正格子點, 則我們有
pn = qm 且
1![]() m
m![]() (p - 1)/2. 然而由 pn = qm 可得 p| qm, 再因
p, q 為相異質數故由 Proposition 1.2.7(1) 知 p| m, 此和
1
(p - 1)/2. 然而由 pn = qm 可得 p| qm, 再因
p, q 為相異質數故由 Proposition 1.2.7(1) 知 p| m, 此和
1![]() m
m![]() (p - 1)/2 相矛盾. 故知在 T1 和 T2
交界的線段上無正格子點. 因此在 T1 和 T2
上的正格子點數之和恰為在 T 上的正格子點數, 故得證
(p - 1)/2 相矛盾. 故知在 T1 和 T2
交界的線段上無正格子點. 因此在 T1 和 T2
上的正格子點數之和恰為在 T 上的正格子點數, 故得證
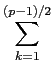
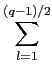
當 p, q 為相異奇質數, 若
M = ![]() [kq/p] 且
N =
[kq/p] 且
N = ![]() [lp/q] 由 Lemma 5.4.4 知
[lp/q] 由 Lemma 5.4.4 知
![]()
![]()
![]() = (- 1)M 且
= (- 1)M 且
![]()
![]()
![]() = (- 1)N. 而 Lemma 5.4.5
告訴我們
M + N = (p - 1)(q - 1)/4, 故得
= (- 1)N. 而 Lemma 5.4.5
告訴我們
M + N = (p - 1)(q - 1)/4, 故得

假設 p = 4k - 1 且 q = 4k' - 1 其中
k, k' ![]()
![]() (即
p
(即
p ![]() q
q ![]() - 1(mod 4)). 則
(p - 1)/2 = 2k - 1 且
(q - 1)/2 = 2k' - 1, 故得
- 1(mod 4)). 則
(p - 1)/2 = 2k - 1 且
(q - 1)/2 = 2k' - 1, 故得
剩下的情況為 p 和 q 中至少有一個在 modulo 4 之後餘 1.
不失一般性就假設
p ![]() 1(mod 4). 此時 p = 4k + 1, 其中
k
1(mod 4). 此時 p = 4k + 1, 其中
k ![]()
![]() ,
故得
(p - 1)/2 = 2k. 而 (q - 1)/2 必為整數故知
,
故得
(p - 1)/2 = 2k. 而 (q - 1)/2 必為整數故知
要注意 Theorem 5.4.6 要在 p, q 為相異奇質數時才適用, 否則若 q
不是奇質數,
![]()
![]()
![]() 這個符號是沒有定義的. 雖然 Theorem 5.4.6
並沒有明確告訴我們
這個符號是沒有定義的. 雖然 Theorem 5.4.6
並沒有明確告訴我們
![]()
![]()
![]() 之值為何, 但是可利用
之值為何, 但是可利用
![]()
![]()
![]() 之值來求得
之值來求得
![]()
![]()
![]() . 一般來說將
. 一般來說將
![]()
![]()
![]() 的問題反轉成
的問題反轉成
![]()
![]()
![]() 的問題就像輾轉相除法一樣, 可以快速的將問題簡化.
這是因為一般來說利用 Lemma 5.3.2(2), 要求
的問題就像輾轉相除法一樣, 可以快速的將問題簡化.
這是因為一般來說利用 Lemma 5.3.2(2), 要求
![]()
![]()
![]() 時,
可假設 q < p, 所以一反轉成
時,
可假設 q < p, 所以一反轉成
![]()
![]()
![]() 時我們已將一個 modulo
比較大的 p 的問題簡化成一個 modulo 比較小的 q 的問題. 例如求
時我們已將一個 modulo
比較大的 p 的問題簡化成一個 modulo 比較小的 q 的問題. 例如求
![]()
![]()
![]() , 由於
101
, 由於
101 ![]() 1(mod 4), 故得
1(mod 4), 故得
![]()
![]()
![]() =
= ![]()
![]()
![]() . 所以馬上將 modulo 101 的問題轉成
modulo 7 的問題, 自然變得簡單. 事實上
. 所以馬上將 modulo 101 的問題轉成
modulo 7 的問題, 自然變得簡單. 事實上
![]()
![]()
![]() =
= ![]()
![]()
![]() ,
可馬上驗證知
,
可馬上驗證知
![]()
![]()
![]() = - 1 (或再用一次 Theorem 5.4.6 得
= - 1 (或再用一次 Theorem 5.4.6 得
![]()
![]()
![]() = -
= - ![]()
![]()
![]() = -
= - ![]()
![]()
![]() = - 1). 所以得知
= - 1). 所以得知
![]()
![]()
![]() = - 1. 總而言之, 對於一般的相異奇質數 p, q,
我們沒有辦法由 p 和 q 馬上得知
= - 1. 總而言之, 對於一般的相異奇質數 p, q,
我們沒有辦法由 p 和 q 馬上得知
![]()
![]()
![]() 之值. 但是利用
Theorem 5.4.6, 我們可以很快速的將問題化簡而求出其值.
最後我們來看一個例子整合這一節中學到的方法.
之值. 但是利用
Theorem 5.4.6, 我們可以很快速的將問題化簡而求出其值.
最後我們來看一個例子整合這一節中學到的方法.
當然了當初若看出
539 = 72×11, 則馬上得
![]()
![]()
![]() =
= ![]()
![]()
![]()
![]()
![]()
![]() =
= ![]()
![]()
![]() . 再因
631
. 再因
631 ![]() 11
11 ![]() 3(mod 4) 以及
631
3(mod 4) 以及
631 ![]() 4(mod 11) 知
4(mod 11) 知