
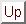

§U§@≠∂: Roots of Polynomials
§W§@≠∂: §§Ø≈ Field ™∫© ΩË
´e§@≠∂: Algebraic Elements
∑Ì F ¨O§@≠” field, L ¨O F ™∫§@≠” extension Æ…, ß⁄≠Õi•H±N L
§§™∫§∏Ø¿§¿¶® algebraic over F ©M§£¨O algebraic over F ™∫®‚∫ÿ.
¶b≥o§@∏`§§ß⁄≠ñN±¥∞Q L §§©“¶≥ algebraic over F ™∫§∏Ø¿©“¶®§ß∂∞¶X.
Definition 10.2.1
∞≤≥]
F ¨O§@≠” field •B
L ¨O
F ™∫§@≠” extension. ß⁄≠ÕO
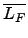
= {
a 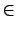 L
L |
a ¨O algebraic over
F},
∫Ÿ§ß¨∞
F ¶b
L ™∫
algebraic closure.
F §§™∫§∏Ø¿∑̵M¨O algebraic over F, ©“•H•—©w∏q™æ
F 

 L. •t• ¶p™G L ¨O F ™∫§@≠” finite
extension, ´h•— Lemma 9.4.5 ™æ L §§™∫§∏Ø¿≥£ algebraic over
F, ©“•H¶b≥o≠”∞≤≥]§ß§U
L. •t• ¶p™G L ¨O F ™∫§@≠” finite
extension, ´h•— Lemma 9.4.5 ™æ L §§™∫§∏Ø¿≥£ algebraic over
F, ©“•H¶b≥o≠”∞≤≥]§ß§U
 = L.
= L.
±µ§U®”ß⁄≠Ã≠n√“©˙
 ™∫§@≠”≠´≠n© ΩË, ßY
™∫§@≠”≠´≠n© ΩË, ßY
 ¨O§@≠”
field. ¥´®•§ß, ß⁄≠Ã≠n√“©˙≠Y
a, b
¨O§@≠”
field. ¥´®•§ß, ß⁄≠Ã≠n√“©˙≠Y
a, b 
 , ®‰§§ b
, ®‰§§ b 0, ´h
a - b •H§Œ
a . b-1 ¨“¶b
0, ´h
a - b •H§Œ
a . b-1 ¨“¶b
 §§ (Lemma
9.1.4). ≠n¶p¶Û√“©˙≥o®«§∏Ø¿≥£¨O algebraic over F ©O?
∑̵M§£•iØý•Œß‰ polynomial ™∫§Ë™k, ß⁄≠Õ≤∂∑¬«ßU Theorem
10.1.9. ¶b≥o§ß´eß⁄≠Õ˝±¿ºs§@§U Definition 10.1.6.
§§ (Lemma
9.1.4). ≠n¶p¶Û√“©˙≥o®«§∏Ø¿≥£¨O algebraic over F ©O?
∑̵M§£•iØý•Œß‰ polynomial ™∫§Ë™k, ß⁄≠Õ≤∂∑¬«ßU Theorem
10.1.9. ¶b≥o§ß´eß⁄≠Õ˝±¿ºs§@§U Definition 10.1.6.
Definition 10.2.2
∞≤≥]
F ¨O§@≠” field •B
L ¨O
F ™∫§@≠” extension. ≠Y
a1,...,
an  L
L, ´h©w
F(
a1,...,
an) ™Ì•Ð¨∞
L §§•]ßt
F
•H§Œ
a1,...,
an ≥çp™∫ field.
Lemma 10.2.3
∞≤≥]
F ¨O§@≠” field •B
L ¨O
F ™∫§@≠” extension. ≠Y
a1,...,
an  L
L ¨“¨∞ algebraic over
F, ´h
F(
a1,...,
an)
¨O
F ™∫§@≠” finite extension. ®∆πͧW, ¶p™G§w™æ
a1,...,
an
over
F ™∫ degree §¿ßO¨∞
m1,...,
mn, ´h
[
F(
a1,...,
an) :
F]
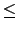 m1 ... mn
m1 ... mn.
µ˝ ©˙.
¨∞§F§Ë´K, ß⁄≠ÕO
K1 = F(a1), K2 = K1(a2) = F(a1, a2),..., Kn = Kn - 1(an) = F(a1,..., an).
πÔ•Ù∑N™∫
i, ß⁄≠ö≥
[
Ki :
Ki - 1] = [
Ki - 1(
ai) :
Ki]
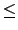 mi
mi. ≥o∏Ã
[
Ki - 1(
ai) :
Ki - 1] ∑|§p©Û©Œµ•©Û
mi ™∫≠϶]¨O: •— Corollary
10.1.7 ™æ
[
Ki - 1(
ai) :
Ki - 1] ™∫≠»≠˶n¨O
ai over
Ki - 1 ™∫ minimal polynomial
qi(
x)
 Ki - 1
Ki - 1[
x] ™∫ degree.
µM¶”•—∞≤≥]
ai over
F ™∫ minimal polynomial
pi(
x)
 F
F[
x] ™∫
degree ¨∞
mi. •—©Û
pi(
x)
 F
F[
x]
 Ki - 1
Ki - 1[
x] •B
pi(
ai) = 0, ¨G•—
qi(
x) ¨O
ai over
Ki - 1 ™∫ minimal
polynomial ™∫∞≤≥]™æ
deg(
qi(
x))
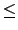
deg(
pi(
x)) =
mi. ¨G™æ
[
Ki :
Ki - 1] = [
Ki - 1(
ai) :
Ki - 1] = deg(
qi(
x))
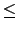 mi
mi.
≤¶b•—©Û®C§@¨q
[Ki : Ki - 1] ≥£¨O¶≥≠≠™∫, ©“•Hß⁄≠Õi•H≥sƒÚÆM•Œ
Theorem 9.4.6 ±o:
| [F(a1,..., an) : F] |
= |
[Kn : Kn - 1][Kn - 1 : F] |
|
| |
= |
[Kn : Kn - 1][Kn - 1 : Kn - 2][Kn - 2 : F] |
|
| |
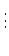 |
|
|
| |
= |
[Kn : Kn - 1] ... [K1 : F]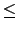 mn ... m1. mn ... m1. |
|
¨G±o√“
F(
a1,...,
an) ¨O
F ™∫§@≠” finite
extension.

ßQ•Œ Lemma 10.2.3 ß⁄≠Ã∞®§W•i±o™æ
 ¨O§@≠” field.
¨O§@≠” field.
Theorem 10.2.4
∞≤≥]
F ¨O§@≠” field •B
L ¨O
F ™∫§@≠” extension. ≠Y
a,
b  L
L,
®‰§§
b
0, ¨“¨∞ algebraic over
F, ´h
a +
b,
a -
b,
a . b
•H§Œ
a . b-1 ¨“¨∞ algebraic over
F. •—¶πß⁄≠Õi±o

¨O§@≠” field.
µ˝ ©˙.
•— Lemma
10.2.3 ß⁄≠Ùæ
F(
a,
b) ¨O
F ™∫§@≠” finite extension.
•—©Û
a,
b  F
F(
a,
b),
b
0 •B
F(
a,
b) ¨O§@≠” field, ß⁄≠ö€µM¶≥
a +
b,
a -
b,
a . b •H§Œ
a . b-1 ¨“¨∞
F(
a,
b) ™∫§∏Ø¿.
¨G•— Theorem
10.1.9 (©Œ Lemma
10.1.3) ™æ≥o•|≠”§∏Ø¿¨“¨∞
algebraic over
F.
§µ≠Y
a, b 
 , ®‰§§ b
, ®‰§§ b 0, ´h•—©w∏q™æ a, b ¨“¨∞
algebraic over F. ¨G•—´e™æ a + b, a - b, a . b •H§Œ
a . b-1 ¨“¨∞ algebraic over F. ¨G™æ≥o•|≠”§∏Ø¿¨“¶b
0, ´h•—©w∏q™æ a, b ¨“¨∞
algebraic over F. ¨G•—´e™æ a + b, a - b, a . b •H§Œ
a . b-1 ¨“¨∞ algebraic over F. ¨G™æ≥o•|≠”§∏Ø¿¨“¶b
 §§,
¶]¶π±o√“
§§,
¶]¶π±o√“
 ¨O§@≠” field.
¨O§@≠” field.

∞≤≥] L ¨O F ™∫§@≠” extension, •B K ¨O L over F ™∫
subextension (ßY
F  K
K  L). L §§¨O algebraic over
K ™∫§∏Ø¿•º•≤¨O algebraic over F. §£πL L §§¨O algebraic over
F ™∫§∏Ø¿¥N§@©w¨O algebraic over K. ≥o¨O¶]¨∞≠Y
a
L). L §§¨O algebraic over
K ™∫§∏Ø¿•º•≤¨O algebraic over F. §£πL L §§¨O algebraic over
F ™∫§∏Ø¿¥N§@©w¨O algebraic over K. ≥o¨O¶]¨∞≠Y
a 
 (ßY a
(ßY a  L ¨O algebraic over F), ™Ì•Ð¶b F[x] §§¶s¶b f (x)
L ¨O algebraic over F), ™Ì•Ð¶b F[x] §§¶s¶b f (x) 0 ®œ±o f (a) = 0. •—©Û
f (x)
0 ®œ±o f (a) = 0. •—©Û
f (x)  F[x]
F[x]  K[x], ß⁄≠ö€µM±o a
§]¨O algebraic over K. ¨G±o
a
K[x], ß⁄≠ö€µM±o a
§]¨O algebraic over K. ¨G±o
a 
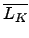 , ¥´•y∏Ъ°ß⁄≠á`¨O¶≥
, ¥´•y∏Ъ°ß⁄≠á`¨O¶≥
ß⁄≠ö≥ø≥ΩÏ™æπD§∞ªÚÆ…≠‘
 ∑|µ•©Û
∑|µ•©Û
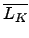 . •H§U¨O§@≠”®“§l.
. •H§U¨O§@≠”®“§l.
Lemma 10.2.5
∞≤≥]
F ¨O§@≠” field,
L ¨O
F ™∫§@≠” extension, •B
K ¨O
L
over
F ™∫ subextension. ≠Y
K ¨O
F ™∫§@≠” finite extension, ´h

=
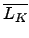
µ˝ ©˙.
ß⁄≠çw™æ


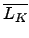
, ©“•H•u≠n√“©˙
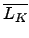


. §]¥N¨O≠n√“©˙: ≠Y
a  L
L ¨O algebraic
over
K, ´h
a ¨O algebraic over
F. ß⁄≠ö“º
K(
a) ≥o§@≠”
field. •—∞≤≥]
a ¨O algebraic over
K, ¨GßQ•Œ Corollary
10.1.7
™æ
K(
a) ¨O
K ™∫§@≠” finite extension. ¶A•[§W
K ¨O
F ™∫§@≠”
finite extension, ÆM•Œ Theorem
9.4.6 •i±o
[K(a) : F] = [K(a) : K][K : F],
¶]¶π
K(
a) ¨O
F ™∫§@≠” finite extension. ¨GßQ•Œ
a  K
K(
a) •H§Œ Theorem
10.1.9 (©Œ Lemma
10.1.3) ™æ
a ¨O
algebraic over
F.

ß⁄≠Õi•H±N Lemma 10.2.5 ±¿ºs®ÏßÛ§@ØΙ∫™¨™p. ¶^≈U§@§U≠Y K
¨O F ™∫§@≠” algebraic extension ™Ì•Ð K §§™∫§∏Ø¿¨“¨∞ algebraic
over F. ¶b Lemma 10.2.5 §§™∫∞≤≥] K ¨O F ™∫ finite
extension, ©“•H¶€µM¨O F ™∫§@≠” algebraic extension. ß⁄≠Ã≠n±N Lemma
10.2.5 ±¿ºs®Ï K ¨O F ™∫ algebraic extension ≥o≠”™¨™p.
Theorem 10.2.6
∞≤≥]
F ¨O§@≠” field,
L ¨O
F ™∫§@≠” extension, •B
K ¨O
L
over
F ™∫ subextension. ≠Y
K ¨O
F ™∫§@≠” algebraic extension,
´h

=
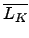
µ˝ ©˙.
©M Lemma
10.2.5 ¨€¶P™∫±°ßŒ, ß⁄≠Õu≠n√“©˙: ≠Y
a  L
L ¨O
algebraic over
K, ´h
a ¨O algebraic over
F.
§£πL≥o∏Ã∏I®Ï™∫™¨™p¨O
K •iØý§£¨O finite extension over
F,
©“•Hß⁄≠ç£Øý™Ω±µÆM•Œ Lemma
10.2.5. ≠nßJ™A≥o≠”ßx√¯,
ß⁄≠Õ≤∂∑∑QøÏ™k߉®Ï§@≠”
F ™∫ finite extension
K' •B∫°®¨
a ¨O
algebraic over
K'. ¶p¶π¶AÆM•Œ Lemma
10.2.5 ±o√“
a ¨O
algebraic over
F.
•—∞≤≥] a ¨O algebraic over K, ™æ¶s¶b f (x) 0 •B
f (x)
0 •B
f (x)  K[x] ®œ±o f (a) = 0. ∞≤≥]
f (x) = anxn + ... + a0. •—©Û
an,..., a0
K[x] ®œ±o f (a) = 0. ∞≤≥]
f (x) = anxn + ... + a0. •—©Û
an,..., a0  K •B K ¨O F ™∫§@≠” algebraic extension, ¨G™æ
an,..., a0 ¨“¨∞ algebraic over F. •O
K' = F(an,..., a0),
•— Lemma 10.2.3 ™æ K' ¨O F ™∫§@≠” finite extension. ¨GßQ•Œ
Lemma 10.2.5 ™æ
K •B K ¨O F ™∫§@≠” algebraic extension, ¨G™æ
an,..., a0 ¨“¨∞ algebraic over F. •O
K' = F(an,..., a0),
•— Lemma 10.2.3 ™æ K' ¨O F ™∫§@≠” finite extension. ¨GßQ•Œ
Lemma 10.2.5 ™æ
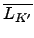 =
=  . •t• •—©Û
an,..., a0
. •t• •—©Û
an,..., a0  F(an,..., a0) = K', ß⁄≠Ùæ
f (x)
F(an,..., a0) = K', ß⁄≠Ùæ
f (x)  K'[x]. ¨G•—
f (a) = 0 ™æ a ¨O algebraic over K'. ¥´®•§ß, ß⁄≠ö≥
a
K'[x]. ¨G•—
f (a) = 0 ™æ a ¨O algebraic over K'. ¥´®•§ß, ß⁄≠ö≥
a 
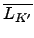 , ¨G•—
, ¨G•—
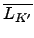 =
=  ±o™æ
a
±o™æ
a 
 .
¶]¶π±o√“ a ¨O algebraic over F.
.
¶]¶π±o√“ a ¨O algebraic over F.

ß⁄≠çw™æ
 ¨O§@≠” filed (Theorem 10.2.4) •B
F
¨O§@≠” filed (Theorem 10.2.4) •B
F 

 L. ¶p™Gß⁄≠öA¶¨∂∞ L §§¨O algebraic
over
L. ¶p™Gß⁄≠öA¶¨∂∞ L §§¨O algebraic
over
 ™∫§∏Ø¿∑|§£∑|±o®ÏßÛ§j™∫ field ™∫©O? ¥´•y∏Ю”ª°,
ß⁄≠Ã∑Q™æπD
™∫§∏Ø¿∑|§£∑|±o®ÏßÛ§j™∫ field ™∫©O? ¥´•y∏Ю”ª°,
ß⁄≠Ã∑Q™æπD
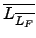 (§£≠n≥Q≥o≤≈∏π¿ µ€§F) ¨O§∞ªÚ?
®∆πͧW©“ø◊™∫ algebraic closure ¥N¨Oª° L §§ algebraic over
(§£≠n≥Q≥o≤≈∏π¿ µ€§F) ¨O§∞ªÚ?
®∆πͧW©“ø◊™∫ algebraic closure ¥N¨Oª° L §§ algebraic over
 ™∫§∏Ø¿©“¶®™∫∂∞¶X¥N¨O
™∫§∏Ø¿©“¶®™∫∂∞¶X¥N¨O
 ¶€§v.
¶€§v.
Corollary 10.2.7
∞≤≥]
F ¨O§@≠” field •B
L ¨O
F ™∫§@≠” extension, ≠Y
a  L
L •B
a ¨O algebraic over

, ´h
a ¨O algebraic over
F.
§]¥N¨Oª°, ß⁄≠ö≥
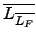
=
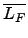
.
µ˝ ©˙.
≠∫•˝™`∑N•—©w∏q

§§™∫§∏Ø¿≥£¨O algebraic over
F, ¨G™æ

¨O
F ™∫§@≠” algebraic extension. ¶]¶π≠Y•O
K =

,
´h
K ≤≈¶X Theorem
10.2.6 ™∫±¯•Û, ¨G™æ
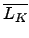
=

.
§]¶]¶π≠Y
a  L
L ¨O algebraic over

=
K, ™Ì•Ð
a 
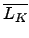
. ¨G•—
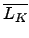
=

±o™æ
a 

,
§]¥N¨Oª°
a ¨O algebraic over
F.



§U§@≠∂: Roots of Polynomials
§W§@≠∂: §§Ø≈ Field ™∫© ΩË
´e§@≠∂: Algebraic Elements
Administrator
2005-06-18
![]()
![]()
![]() L. •t• ¶p™G L ¨O F ™∫§@≠” finite
extension, ´h•— Lemma 9.4.5 ™æ L §§™∫§∏Ø¿≥£ algebraic over
F, ©“•H¶b≥o≠”∞≤≥]§ß§U
L. •t• ¶p™G L ¨O F ™∫§@≠” finite
extension, ´h•— Lemma 9.4.5 ™æ L §§™∫§∏Ø¿≥£ algebraic over
F, ©“•H¶b≥o≠”∞≤≥]§ß§U
![]() = L.
= L.
![]() ™∫§@≠”≠´≠n© ΩË, ßY
™∫§@≠”≠´≠n© ΩË, ßY
![]() ¨O§@≠”
field. ¥´®•§ß, ß⁄≠Ã≠n√“©˙≠Y
a, b
¨O§@≠”
field. ¥´®•§ß, ß⁄≠Ã≠n√“©˙≠Y
a, b ![]()
![]() , ®‰§§ b
, ®‰§§ b![]() 0, ´h
a - b •H§Œ
a . b-1 ¨“¶b
0, ´h
a - b •H§Œ
a . b-1 ¨“¶b
![]() §§ (Lemma
9.1.4). ≠n¶p¶Û√“©˙≥o®«§∏Ø¿≥£¨O algebraic over F ©O?
∑̵M§£•iØý•Œß‰ polynomial ™∫§Ë™k, ß⁄≠Õ≤∂∑¬«ßU Theorem
10.1.9. ¶b≥o§ß´eß⁄≠Õ˝±¿ºs§@§U Definition 10.1.6.
§§ (Lemma
9.1.4). ≠n¶p¶Û√“©˙≥o®«§∏Ø¿≥£¨O algebraic over F ©O?
∑̵M§£•iØý•Œß‰ polynomial ™∫§Ë™k, ß⁄≠Õ≤∂∑¬«ßU Theorem
10.1.9. ¶b≥o§ß´eß⁄≠Õ˝±¿ºs§@§U Definition 10.1.6.
![]() ¨O§@≠” field.
¨O§@≠” field.
![]()
![]() , ®‰§§ b
, ®‰§§ b![]() 0, ´h•—©w∏q™æ a, b ¨“¨∞
algebraic over F. ¨G•—´e™æ a + b, a - b, a . b •H§Œ
a . b-1 ¨“¨∞ algebraic over F. ¨G™æ≥o•|≠”§∏Ø¿¨“¶b
0, ´h•—©w∏q™æ a, b ¨“¨∞
algebraic over F. ¨G•—´e™æ a + b, a - b, a . b •H§Œ
a . b-1 ¨“¨∞ algebraic over F. ¨G™æ≥o•|≠”§∏Ø¿¨“¶b
![]() §§,
¶]¶π±o√“
§§,
¶]¶π±o√“
![]() ¨O§@≠” field.
¨O§@≠” field.
![]()
![]() K
K ![]() L). L §§¨O algebraic over
K ™∫§∏Ø¿•º•≤¨O algebraic over F. §£πL L §§¨O algebraic over
F ™∫§∏Ø¿¥N§@©w¨O algebraic over K. ≥o¨O¶]¨∞≠Y
a
L). L §§¨O algebraic over
K ™∫§∏Ø¿•º•≤¨O algebraic over F. §£πL L §§¨O algebraic over
F ™∫§∏Ø¿¥N§@©w¨O algebraic over K. ≥o¨O¶]¨∞≠Y
a ![]()
![]() (ßY a
(ßY a ![]() L ¨O algebraic over F), ™Ì•Ð¶b F[x] §§¶s¶b f (x)
L ¨O algebraic over F), ™Ì•Ð¶b F[x] §§¶s¶b f (x)![]() 0 ®œ±o f (a) = 0. •—©Û
f (x)
0 ®œ±o f (a) = 0. •—©Û
f (x) ![]() F[x]
F[x] ![]() K[x], ß⁄≠ö€µM±o a
§]¨O algebraic over K. ¨G±o
a
K[x], ß⁄≠ö€µM±o a
§]¨O algebraic over K. ¨G±o
a ![]()
![]() , ¥´•y∏Ъ°ß⁄≠á`¨O¶≥
, ¥´•y∏Ъ°ß⁄≠á`¨O¶≥
![]() 0 •B
f (x)
0 •B
f (x) ![]() K[x] ®œ±o f (a) = 0. ∞≤≥]
f (x) = anxn + ... + a0. •—©Û
an,..., a0
K[x] ®œ±o f (a) = 0. ∞≤≥]
f (x) = anxn + ... + a0. •—©Û
an,..., a0 ![]() K •B K ¨O F ™∫§@≠” algebraic extension, ¨G™æ
an,..., a0 ¨“¨∞ algebraic over F. •O
K' = F(an,..., a0),
•— Lemma 10.2.3 ™æ K' ¨O F ™∫§@≠” finite extension. ¨GßQ•Œ
Lemma 10.2.5 ™æ
K •B K ¨O F ™∫§@≠” algebraic extension, ¨G™æ
an,..., a0 ¨“¨∞ algebraic over F. •O
K' = F(an,..., a0),
•— Lemma 10.2.3 ™æ K' ¨O F ™∫§@≠” finite extension. ¨GßQ•Œ
Lemma 10.2.5 ™æ
![]() =
= ![]() . •t• •—©Û
an,..., a0
. •t• •—©Û
an,..., a0 ![]() F(an,..., a0) = K', ß⁄≠Ùæ
f (x)
F(an,..., a0) = K', ß⁄≠Ùæ
f (x) ![]() K'[x]. ¨G•—
f (a) = 0 ™æ a ¨O algebraic over K'. ¥´®•§ß, ß⁄≠ö≥
a
K'[x]. ¨G•—
f (a) = 0 ™æ a ¨O algebraic over K'. ¥´®•§ß, ß⁄≠ö≥
a ![]()
![]() , ¨G•—
, ¨G•—
![]() =
= ![]() ±o™æ
a
±o™æ
a ![]()
![]() .
¶]¶π±o√“ a ¨O algebraic over F.
.
¶]¶π±o√“ a ¨O algebraic over F.
![]()
![]() ¨O§@≠” filed (Theorem 10.2.4) •B
F
¨O§@≠” filed (Theorem 10.2.4) •B
F ![]()
![]()
![]() L. ¶p™Gß⁄≠öA¶¨∂∞ L §§¨O algebraic
over
L. ¶p™Gß⁄≠öA¶¨∂∞ L §§¨O algebraic
over
![]() ™∫§∏Ø¿∑|§£∑|±o®ÏßÛ§j™∫ field ™∫©O? ¥´•y∏Ю”ª°,
ß⁄≠Ã∑Q™æπD
™∫§∏Ø¿∑|§£∑|±o®ÏßÛ§j™∫ field ™∫©O? ¥´•y∏Ю”ª°,
ß⁄≠Ã∑Q™æπD
![]() (§£≠n≥Q≥o≤≈∏π¿ µ€§F) ¨O§∞ªÚ?
®∆πͧW©“ø◊™∫ algebraic closure ¥N¨Oª° L §§ algebraic over
(§£≠n≥Q≥o≤≈∏π¿ µ€§F) ¨O§∞ªÚ?
®∆πͧW©“ø◊™∫ algebraic closure ¥N¨Oª° L §§ algebraic over
![]() ™∫§∏Ø¿©“¶®™∫∂∞¶X¥N¨O
™∫§∏Ø¿©“¶®™∫∂∞¶X¥N¨O
![]() ¶€§v.
¶€§v.